Grand Jeu d'Hiver TSM 2007-2008

en considere Un=1+1/1!+...1/n!
f(x)=e(-x)*(1+x/1!+x²/2!...x^n/(n!))
f'(x)=-e(-x)*(1+x/1!+x²/2!...x^n/(n!))+e(-x)*((1+x/1!+x²/2!...x^n/(n!))')
=-e(-x)*(1+x/1!+x²/2!...x^n/(n!))+e(-x)*(0+1+x/1!..x^'n-1)/(n-1)!)=-e(-x)*(x^n/n!)>-x^n/n!
donc pour tt x£[0;1] -1/n!<=f'(x)<=0
avec TAF ily'a c£[0;1] f'(c)=(f(1)-f(0))/(1-0)=e(-1)*Un-1
et puisque c£[0;1] donc -1/(n!)<=f'(c)=<0
donc -1/n!<=e(-1)*Un-1<=0 ===>lime(-1)*Un-1=0
limUn=e

alez poste ton exo mohamed

mohamed_01_01 a écrit:

en considere Un=1+1/1!+...1/n!
f(x)=e(-x)*(1+x/1!+x²/2!...x^n/(n!))
f'(x)=-e(-x)*(1+x/1!+x²/2!...x^n/(n!))+e(-x)*((1+x/1!+x²/2!...x^n/(n!))')
=-e(-x)*(1+x/1!+x²/2!...x^n/(n!))+e(-x)*(0+1+x/1!..x^'n-1)/(n-1)!)=-e(-x)*(x^n/n!)>-x^n/n!
donc pour tt x£[0;1] -1/n!<=f'(x)<=0
avec TAF ily'a c£[0;1] f'(c)=(f(1)-f(0))/(1-0)=e(-1)*Un-1
et puisque c£[0;1] donc -1/(n!)<=f'(c)=<0
donc -1/n!<=e(-1)*Un-1<=0 ===>lime(-1)*Un-1=0
limUn=e

comment a deives la fonction 1/n! §§§§!!!://????????????????

j'ai pas derivie 1/n!

mohamed_01_01 a écrit:

j'ai pas derivie 1/n!

mais t as derives la somme de x/k!

f'(x)=-e(-x)*(1+x/1!+x²/2!...x^n/(n!))+e(-x)*((1+x/1!+x²/2!...x^n/(n!))')

f'(x)=(e(-x)'*(1+x/1!+x²/2!...x^n/(n!))+e(-x)(1+x/1!+x²/2!...x^n/(n!)')
=-e(-x)(1+x/1!+x²/2!...x^n/(n!))+e(-x)((1)'+(x/1!)'+(x²/2!)'...(x^n/(n!)')
et pour tt k#0 (x^k/k!)'=(x^k)'/k!=k*x^(k-1)/k!=x^(k-1)/(k-1)! d'ou la resultat

badr a écrit:

mohamed_01_01 a écrit:

j'ai pas derivie 1/n!

mais t as derives la somme de x/k!

f'(x)=-e(-x)*(1+x/1!+x²/2!...x^n/(n!))+e(-x)*((1+x/1!+x²/2!...x^n/(n!))')

poui j'avais deriver x^k/k! et l'inconnue ici c'est x c'est pas k
(x^k/k!)=(x^k)'/k!=(k*x^(x-1)/k!)=x^(k-1)/(k-1)!

demontrer que
cos(x^3)+cos(y^3)+cos(z^3)+cos(xy)+cos(xz)+cos(zy)<6

on A=cos(x3)+cos(y3)+cos(z3)+cos(xy)+càs(yz)+cos(xz)=<6

donc on doit etudier le cas d'egalite A=6

donc tout les valeurs doivent etre maxi
x^3=2kp et y^3=2np et z^3=2mp et aussi xy=2sp et yz=2tp et zx=2lp avec(k n m s t l) appartiennetà (z)

ona x^3y^3=4knp^2=8s^3p^3 alors kn=2ps^3 cqui est impossible car kn appartient à (z) de la meme façon on demontre les autres donc on deduire que Atokhalife6

donc A<6

oui MRS abdeltif mais tu n'as pas le droit de repondre tu n'est pas inscrit et je n'accepte pas ton inscription lol
tu dois demande à omar
A+ singe

iwa si mohhamed ana ma3arefche
iwa dis moi comment je vais inscrir

iwa si la coste qu' as dis tu

tu dois le demande à omar Mrs singe

abdellatif90 a écrit:

iyehe asi la coste tantla9a m3ake nwirike

Abdeltif est déjà inscrit Maintenant ! Mohamed avait raison.
Ben maintenant Abdeltif Poste ton Exercice .

merci omar

soit a et b min R*+ montrer que

rac(a+b)*(1/rac(a)+1/rac(b))>=2rac2

rac(a+b)>=rac(2)(rac(a)+rac(b))/2
rac(a)+rac(b)(1/rac(a) + 1/rac(b))>=4 (C-S)
d ou le resultats

rac(a+b)>=rac2*rac(rac(ab))
et 1/raca+1/racb>=2rac(1/rac(ab))
d'ou rac(a+b)*(1/rac(a)+1/rac(b))>=2rac2
@++

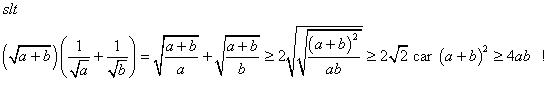Puisque Stof tu es le premier a postée la solution alors Poste ton exercice?

slt omar cmt tu fais pour poster tes solution comme ça

allez stof poste ton exercice

est ce que je peut participer, svp (nouveau membre)

wi tu peu mais tu dois demander ça à alauoi-omar

abdellatif90 a écrit:

wi tu peu mais tu dois demander ça à alauoi-omar

merci

this exercise is in Top math

je veux participer SVP

puis tous ne voulez postez un exo

je profitr la chance pour poster cette exo

{U_a donne
{U_n+1=7U_n+12/(U_n+3)

touvez l'expression de U_n